Matlab: Cone/Arrow in 3D

Cone/Arrow in 3D

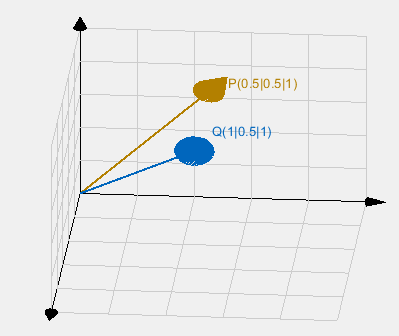
To plot a cone or arrow in 3D you can use this function:

function arrow3D(startVec, stopVec, varargin)

The startVec is the beginning of the 3D cone and the stopVec the end of the cone. Ypi can use the optional arguments as 'Color','Thickness','Length' to customize your cone.

Source code:

function arrow3D(startVec,stopVec,varargin)

% Input arguments:

% startVec ... input vector of the beginning of the point in [x,y,z]

% stopVec ... input vector of the ending of the point in [x,y,z]

%

% Optional input argument (varargin):

% 'Color',color ... define the color of the arrow/point

% 'Thickness',thick ... thickness of the arrow

% 'Length',length ... Length of the arrow

% 'Axes',ax ... Input axes

    if isempty(varargin)

        varargin{1} = '';

    end

    

    [x] = startVec(1); 

    [y] = startVec(2); 

    [z] = startVec(3); 

    x2 = stopVec(1);

    y2 = stopVec(2);

    z2 = stopVec(3);

    

    [logic, index] = max(strcmp(varargin,'Length'));

    if logic

        length = varargin{index+1};

    else

        length = mean(abs(stopVec-startVec))/10;

    end

    

    [logic, index] = max(strcmp(varargin,'Axes'));

    if logic

        ax = varargin{index+1};

    else

        ax = gca;

    end

    

    [logic, index] = max(strcmp(varargin,'Thickness'));

    if logic

        thick = varargin{index+1};

    else

        thick = mean(abs(stopVec-startVec))/10;

    end

    

    [logic, index] = max(strcmp(varargin,'Color'));

    if logic

        color = varargin{index+1};

    else

        color = [0 0 0];

    end

    

    vector2=[x2;y2;z2];

    vector1=[x;y;z];

    vector21=[x2-x;y2-y;z2-z];

    u1=[x2-x;y2-y;z2-z];

    u1=1/norm(u1)*u1;

    u2=[1;0;0];

    val=u1(1,1);

    abstand=u1-val*u2;

    if abstand==zeros(3,1)

        u2=[0;1;0];

    end

    

    %Kreuzprodukt

    u3(1,1)=u1(2,1)*u2(3,1)-u1(3,1)*u2(2,1);

    u3(2,1)=u1(3,1)*u2(1,1)-u1(1,1)*u2(3,1);

    u3(3,1)=u1(1,1)*u2(2,1)-u1(2,1)*u2(1,1);

    u3=1/norm(u3)*u3;

    %

    u2(1,1)=u1(2,1)*u3(3,1)-u1(3,1)*u3(2,1);

    u2(2,1)=u1(3,1)*u3(1,1)-u1(1,1)*u3(3,1);

    u2(3,1)=u1(1,1)*u3(2,1)-u1(2,1)*u3(1,1);

    u2=1/norm(u2)*u2;

    %orthnormale vektoren

    v1=u2;

    v2=u3;

    step=pi/50;

    a = step:step:2*pi;

    er=vector2;

    Er=repmat(er,1,numel(a));

    zerx=(Er(1,:))';

    zery=(Er(2,:))';

    zerz=(Er(3,:))';

    vectorscale=(1-length)*vector21;

    grund=(v1*sin(a)+v2*cos(a))*norm(vector2'-vector1')/3*thick;

    VS=repmat(vectorscale+vector1,1,numel(a));

    grund=grund+VS;

    xspitz=[zerx';grund(1,:)];

    yspitz=[zery';grund(2,:)];

    zspitz=[zerz';grund(3,:)];

    xrep=reshape(xspitz,1,2*numel(a))';

    yrep=reshape(yspitz,1,2*numel(a))';

    zrep=reshape(zspitz,1,2*numel(a))';

    plot3(ax, xrep, yrep, zrep, 'Color', color);

end